203: Gotta be a fraction, right?

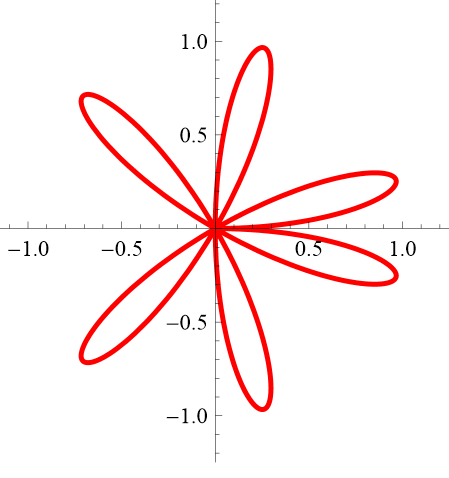
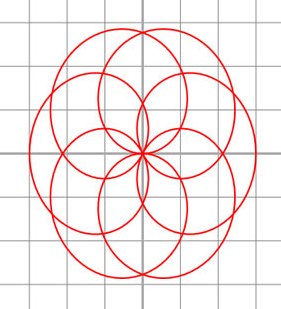
Is this thing rational?

202: What is the area?

What combinations of addition or subtraction of figures could you use to find the area of the white?

201: Circles and Pentagon

Two circles, radius 1 (each does not pass through the others center).
All seven regions are of equal area.
What is the area of the pentagon?

200: IQ and College Major

This should get everyone arguing.

199: What is that shape?

Is this one shape or three?

198: Packing tires

Given the following information, what's the best packing method for those tires in one of these containers? You might want to Desmos-Draw the floorplan to be sure.

.

Overall diameter is 25", BTW.

197: Polar Figures 6

Can you recreate these figures? Note: Unlike the previous figures, these are all "incomplete" so you will have to do two things ... (a) figure out the form of the full figure (how many pedals, etc.) and (b) restrict the domain appropriately.  In Desmos, domain restriction is done this way:

Here we go ....

196: Polar Figures 5

Can you recreate these figures?

This family ...

And this single function

195: Polar Figures 4

Can you recreate these figures?

194: Polar Figures 3

Can you recreate these figures?

Single function.

In Desmos or Plot.ly, Create this Family of functions.

193: Polar Figures 2

Can you recreate these figures?

This last one is a piece-wise function ...

192: Polar Figures 1

Can you recreate these figures?

191: Walking the Labyrinth

In this labyrinth, there are no false turns or dead ends. You walk the line around the outside, then follow it to the middle. Assume unit distance between lines and ends of turns except for that horizontal entry line. That seems to be half a unit apart from the other lines.

What are some different ways to measure the distance walked?

190: Force Table

If the weights are all equal masses, is this in equilibrium?

If not, how would you change it?
Can this arrangement work with two equal masses?

189: Strasbourg Cathedral

Find the visual fractions.
Discuss the geometrical constructions the builder would have employed if he were limited to the following tools: a straight iron rod of 16.5 feet, string and a piece of chalk.

188: XKCD Check

So ... how much did Munroe actually pay to Verizon?

187: Spiral

If we kept going, would any of the sides in this figure be parallel?

UPDATE: Asking a bad question is my fault. Of course, any polygon that has an even number of sides has n/2 pairs of parallel sides. What I was originally after, but didn't ask properly, was whether there a side of one polygon that was parallel to a side in another ... other than the pair in the triangle and square.

186: Candle, Candle, Burning Bright

How long has this candle stand been in that corner?

185: Four is Cosmic

8 is 5; 5 is 4 ... and 4 is cosmic.
9 is 4 ... and 4 is cosmic.
10 is 3, 3 is 5, 5 is 4 ... and 4 is cosmic.
17 is 9, 9 is 4 ... and 4 is cosmic.
37 is 11, 11 is 6, 6 is 3, 3 is 5, 5 is 4 ... and 4 is cosmic.

What is going on?
Does everything lead to four?

184: Is Eight Magic?

What is the pattern if we do this for other two-digit numbers?

Can we get other results? Are there results that we CAN'T get?
Challenge:
   What is the longest string?

183: Italian Roulette

Could this work? What might go wrong with this plan?

If you divided the pizza into EIGHT pieces, what would be your chances of having to pay?

182: Inscribed Pyramid 3

How can we find the volume of the pyramid?

181: Inscribed Pyramid 2

If each RED line segment has a length of 10 cm, what shape is the black object?
How would we go about finding its volume?

red = 10 cm

180: Inscribed Pyramid 1

If each black line segment has a length of 10 cm, how can we find the volume of the pyramid?

black = 10 cm

What is the general rule for this type of thing?

179: How Far to Bill's House?

Which distance is shorter?

Challenge:

Which two houses are the furthest apart when traveling by road?

178: Hexagonal Densepack

What is the best way to find the perimeter of the rounded hexagonal shape, i.e., the length of the belt that would wrap around the six wheels?

r = 1

177: Parabola and friends

Is it possible to choose four points on the graph of y = x² that are the vertices of a trapezoid?
A parallelogram?
An equilateral triangle?

Here's y = x² to help you think.

Is it possible to choose four pts on the graph of y = x² that are the vertices of a trapezoid? A parallelogram? An equilateral triangle?
— James Tanton (@jamestanton)

176: Reported Ages

Sayeth Mr Honner:

Here’s a fun little data set from a statistics textbook I’m reading.



These are the distributions of last digits of ages reported on the 1880 and 1970 US Censuses. At least two interesting questions come to mind, one with a seemingly easy answer.

What do you think his two questions are?

What other questions did you have?

What would the graph look like if we asked people for the ages of their parents?

175: Analyze This!

How many of the causation rules does this graph violate?

174: Factors and Multiples

Least Common Multiple and Greatest Common Factor

A, B, and C are positive integers.

Greatest Common Factor of A and B is 5
Greatest Common Factor of B and C is 2
Greatest Common Factor of C and A is 3
Least Common Multiple of A and B is 900
Least Common Multiple of B and C is 2100
Least Common Multiple of C and A is 630

What is A?

173: Bathtubs

Math is not always as easy for journalists as it is for you. Help this one with some of his calculations and assumptions.